Genetic-Mathematical Modelling of the Populations Interaction

If there is some mutagen factor D_2,1 working from the second population on first population the law of Hardy - Weinberg for the first population it is possible to write down as ^{1, 2}:

.......(1)

Where q _f1 there is frequency of recessive female allele of the first population subjected to influence from the second population. As the first population we consider cells of the person organism for example cells of a liver. Dimensionless time n₁=t/T₁ , where T₁ - average time of a life of organism cells for an individual of the first population. For cells of a liver on different sources is T₁ ≈ 140days ^{3, 4, 5}.

The Hardy - Weinberg law for the second population we shall write down similarly:

......(2)

At writing of the equation (2) we have assumed that in the second population there is no division into male and female individuals, so Q₂ there is a frequency of recessive allele which is subjected to influence in genome of the second population. For example, such situation is characteristic for bacterial, microbic or virus populations. Dimensionless time n₂=t/T₂, where T₂ there is average time of an individual life of the second population. For example, for a virus of flu is T₂≈ 7 days ^{3, 4, 5}. The mutagen factor working on immune system of the first population on second, is designated D_1,2.

Let's enter uniform dimensionless time. From a condition n₁T₁ = n₂T₂ we find n₂=(T₁/T₂)n₁=(1/γ)n₁

where a constant γ = T₂/T₁≈0,05 Hence the equation (2) can be copied as:

…..(3)

Let's examine the mutagen factors. We shall assume that allele in genome of the second population q₂ at interaction with the first population it is inserted in a genome of the first population with catastrophic for a cell of the first population consequences. Hence, it is possible to assume that the mutagen factor is D_2,1=α₁q₂ where α₁<0 there is some constant factor.

Influence of the first population on the second consists in destruction by immune system of the first population of individuals (viruses) of the second population. Hence, it is possible to assume that D_1,2 = α₂, and size α₂<0.

Thus the equations (1) and (3) get a kind:

...(4)

.....(5)

In the equations (4) and (5) index at dimensionless time is omitted since transition by uniform dimensionless time n=n₁ was carried out.

The equation (5) for the second (virus) population at influence on it of the first population immune system can be solved irrelatively of the equations (4).

We integrate once the equation (5):

.....(6)

Where C₁ there is a constant of integration.

For the solution of the equation (6) we shall present function q₂ as product of two functions q₂=uv . Then the equation (6) will be copied as:

…..(7)

Grouping the terms we shall find:

....(8)

Expression in brackets we shall accept equal to zero γdu/dn+ln2u=0. Integrating this equality we shall find lnu=-ln2n/γ=-ln2^n/γ . Hence there is u=2^-n/γ. A constant of integration it is accepted equal to zero since there is some arbitrariness in a choice of function u. Substitute this function in (8) we shall receive:

.....(9)

Solving the equation (9) concerning function V we find:

.....(10)

Hence:

…..(11)

Thus for allele frequency q₂ we shall find:

....(12)

We use the following conditions: at n=0 the allele frequency is q₂ = q₂₀, at n/γ=1/ln2 allele frequency is . The second condition is equivalent to time t=1.443t₂, i.e. the second condition is accepted for time approximately in one and a half time more than average time of an individual life of the second population.

Hence on the first condition:

….(13)

on the second condition:

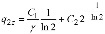….(14)

Solving the system (13), (14) we find:

…...(15)

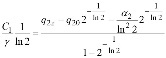….(16)

Substituting (15) and (16) in (12) we shall receive:

……..(17)

The formula (17) can be written down as the sum of two terms: reflecting duplication in the second (virus) population and influence on it first population:

.....(18)

Let's note that duplication in a virus population occurs not independently but only at interaction to cells, for example, a human population. Therefore at the first term (18) there is a size γ=T₂/T₁ dependent on average time of a cell life of the first (human) population. The second term (18) reflects action of the person immune system on the second (virus) population.

On Figure 1 change of an allele frequency q₂ the second (virus) population in time at absence of influence of the person immune system (curve 1), and at presence of influence (curve 2) of the first population immune system is shown.

Figure 1.Change of an allele frequency q2 in time for the second (virus) population at absence of influence of the first (human) population immune system, a curve 1 and at presence of influence, a curve 2. Parameters for calculation q0 =0,3;; q2=0,65; γ=0,05;α2 = -0.01.

From the analysis of graphs it is visible that at absence of influence of a human population immune system on a virus population there is a gradual growth of the allele frequency q₂ in time; the approach of allele frequency q₂ to equilibrium value. At presence of influence of the first (human) population immune system the growth q₂ is replaced by falling due to destruction of viruses at action of immune system of the first population.

The most interesting question it is work of organism cells of a human population at their interaction with viruses. Some results of such interaction can be received at the solution of the equation (4).

The solution of the equation (4) can be received substitution of the solution (18) in the equation (4). However such way is difficult occurrence of complex function in the right part of the equation (4). Therefore we shall increase the order of the equation having substituted function q₂ from the equation (4) in the equation (5). In result we shall receive the linear differential equation of the fourth order:

....(19)

Integrating the equation (19) twice we find:

…(20)

where C₃ and C₄ there are constants of integration.

For the solution of the equation (20) we shall find the general solution of the homogeneous equation. We shall write down the characteristic equation:

…..(21)

Solutions of a quadratic equation (21) look like:

K₁ = -ln2, K₂ = - ln2/γ ….(22)

Hence the general solution of the homogeneous equation looks like:

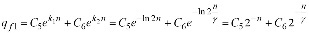…..(23)

Where C₅ and C₆ there are constants of integration.

The particular solution of the equation (20) we shall search as a polynom:

…….(24)

where A, B and D there are constant factors.

Substituting (24) in (20) we shall find:

.....(25)

Equating factors at identical degrees n we find:

......(26)

ConstantD in (24) to find there is no necessity.

Thus the particular solution (24) of equation (20) looks like:

….(27)

Hence the solution of the equation (20) will be written down as the sum (23) and (27):

......(28)

Let's accept the initial condition: at n=0 size is q_f1 = q_f0.

Hence:

q_f0 = C₅ + C₆ + D …….(29)

Thus:

.....(30)

The found solution (30) characterizes the sum of two processes: duplication of individuals of the first population - first three terms, and the influence on a population - the fourth term.

Absence of influence on the first population there are probably in two cases. First, at α₁ = 0 , i.e. the second population does not operate on the first population; second, at α₂ = α₁ = 0 these populations develop independently from each other and thirdly, zero value of expression in brackets of the fourth term (30).

Last case is most interesting. It is connected to a variant when on the first population, for example, human, was influence of the second population, for example, virus, but then it has stopped since the human population has developed immunity to influence of a virus population.

Let's equate last bracket (30) to zero:

.....(31)

The equation (31) allows find dimensionless time of immunity development at the first population to influence of the second population:

…..(32)

Dimensional time is equal:

.....(33)

Unfortunately, the formula (33) has a uncertain constant C₃.

The constant C₃. is defined according to (19) the sum of initial values of first three derivatives on time from the allele frequency q_f1. Integrating (19) once at n=0 we shall find:

……..(34)

Let's assume γ = T₂/T₁ very small size. It is possible since average time of a cell life of the person usually is much greater average time of a virus life. In this case according to (34) a constant is C₃ ≈0.

The formula (30) at C3 ≈0 also γ<<1 looks like:

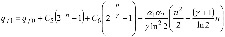.....(35)

At C₃ ≈0 dimensionless time of influence attenuation of the second population on the first population (32) it is equal , n_lmm =2/ln2 ≈ 2,9 dimensional time (33) is equal t_lmm =2/ln2 ≈ 2,9T₁≈400days. Found time of collective immunity occurrence - almost 3 average of time of a cell life of an individual. After the ending of influence of the second population on the first the last term in (35) becomes equal to zero. There is a free duplication in the first population. Taking into account also γ<<1 it is possible to write down:

.....(36)

The law of free duplication of a population looks like ^{1, 2}:

…..(37)

where q_fo there is initial frequency of examined recessive allele at the woman, q_m0 - at the man.

Comparing (36) and (37) we find C₅ = (q_f0 - q_m0)/3. As it was supposed, appeared C₆ = 0, since q_f1 at free duplication of individuals should not depend on size γ. Therefore the formula (35) can be copied as:

…... (38)

On Figure 2 change of an allele frequency q_f1 the first (human) population in time at absence of influence (curve 1), and at presence of influence (curve 2) of the second (virus) population is shown.

Figure 2.Change of the allele frequency qf1 in time at the first (human) population at absence of influence of the second (virus) population (curve 1) and at presence of influence (curve 2). Parameters for calculation qf0 = 0,3; qmo = 0,3; γ = 0,005; α1 = -0.1; α2 =-0.01.

From Figure 2 it is visible that allele frequency at absence of influence of a virus population gradually falls coming nearer to equilibrium value q_f1 = (2q_f0 + q_mo)/3 = 0,23 . At influence of a virus population the allele frequency on which influence is carried out (curve 2) first falls very quickly due to destruction of cells and accordingly alleles but then in process of development of immunity at n_imm = 2/ln2 ≈ 2,9 and duplication of cells the frequency grows reaching the normal level corresponding to a curve 1. The further growth of a curve 2 shown by a dotted line apparently of biological sense has no.